Begin with a set of nine playing cards numbered Ace (representing one) to 9 from any one suit.
The cards are placed face down on the table so that the any one can can be easily picked up at random.
A copy of the diagram (below) showing the positioning of digits in the answer is made on paper. As the cards are selected the challenge is to choose in which cell it will be written. You cannot change your mind after the digit has been written down (and before the next card is chosen).
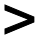
You are successful if: The two digit number on the left is greater than the two digit number on the right
If you are successful your score is the two digit number on the right. Play the game repeatedly keeping a cumulative total of your score.
There are many variations of this game on the main Greater Than page.
You can try similar activities which are online and interactive: Great Expectation
|
|
Do you have any wonderful ideas for using playing cards to help learn mathematics? Please share your ideas here. Playing cards have been around since the ninth century. They were invented in China and spread across Europe in the fourteenth century. Though the designs on the cards have changed over the years the basic number properties have not. Because they are so popular the manufacture of high quality cards is not expensive and makes them an ideal tool for learning mathematics. |
|
SuggestedPrime Pairs Game A game for two players who take turns to select two numbers that add up to a prime number. |
SuggestedSuperior A game for two players who compete to make the largest possible number from randomly selected cards. |
SuggestedManifest An exciting and thought-provoking number placing game for two players or one player versus the computer. |
SuggestedNevertheless Players decide where to place the cards to make an equation with the largest possible solution. |
SuggestedFifteen A strategy game. Play against the computer to select three numbers that add up to 15. |
SuggestedSquare Pairs Game A game for two players who take turns to select two numbers that add up to a square number. |
Ann Mason, Kingsmead School Derby
Tuesday, November 17, 2015
"NUMBER BONDS TO TEN
Remove all 10, j, q, k cards from a pack of cards.
Pupil turns over cards to create 8 separate piles.
Pairs of cards can be covered, with new cards from the pack, if the two numbers add up to 10. (Ace is taken as 1)
The aim is to get rid of all the cards - not always possible, but they soon learn to recognise the number bonds.
Could be timed to create competition as they get more confident."