Sign In | Starter Of The Day | Tablesmaster | Fun Maths | Maths Map | Topics | More
This is the Transum Newsletter for the month of January. It is being published on the first of January when the shorthand version of the date has a Fibonacci feel about it: 1-1-23
I'll begin, as usual with the puzzle of the month.
A teacher writes six words on a board: “cat dog has max dim tag.” She gives three students, Arshad, Billy and Chloe each a piece of paper with one letter from her favourite word in the list (they each have a different letter from the same word). Then she asks, “Arshad, do you know the word?” Arshad immediately replies "yes". She asks, “Billy, do you know the word?” He thinks for a moment and replies "yes". Then she asks Chloe the same question. She thinks and then replies "yes". What is the teacher's favourite word?

The answer and credit for this puzzle will be revealed next month.
While you think about that here are some of the key resources added to the Transum website during the last month.
Pythagoras Level 6 contains a selection of hand-drawn sketches. These were drawn by Dr Tim when he found a need for some Pythagoras questions involving more than one triangle. Tim generously gave me permission to use them as part of the collection of Pythagoras questions. They help provide variety to the exercises and show that even a very simple diagram can be very informative.
A Grade 5 student (equivalent to UK upper Primary) wrote a paper! Yes an academic paper about the RowCol Game. I have included his paper in the comments section of that page and it makes a very interesting read! Well done Noah!
The Place Value exercise now has five levels instead of one. Beyond the standard questions are three tables to be completed in increasing order of difficulty. It is a nice resource adding depth to this topic.
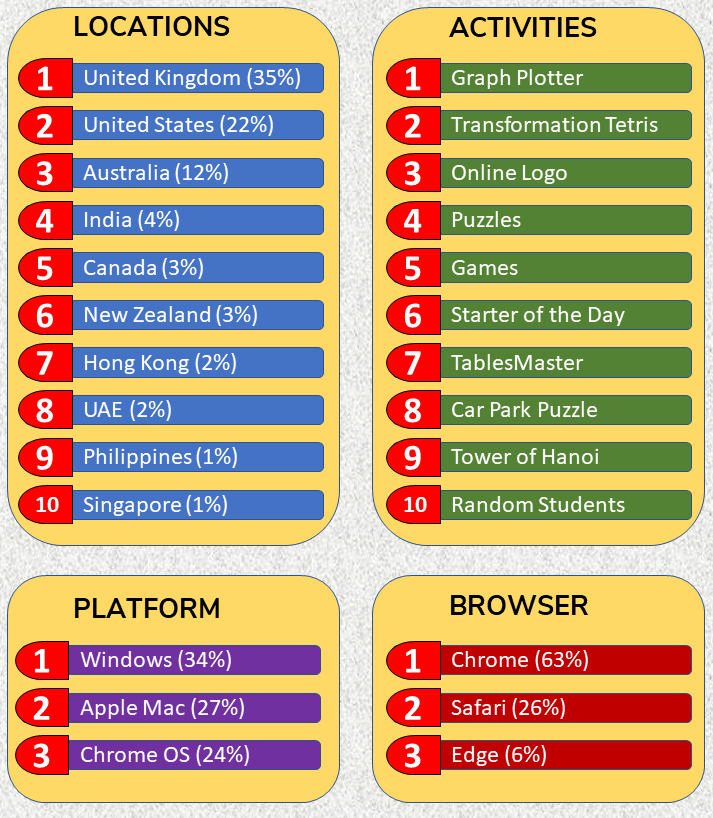
Many publications begin a new year with a look back at the previous year. I thought it would be interesting to share with you some top tens of 2022 (from Google Analytics). Here are the top ranking locations of Transum visitors, the most visited Transum pages and the the top three platforms and browsers:
I think all of you reading this newsletter in early January will be on holiday as of course will your pupils. You probably know that there is a section of the Transum website specifically designed to direct inquisitive minds to mathematical activities that are fun and rewarding to do at home until school reopens. Share this link for School Holiday Maths Activities so that your pupils can try them at home.
In addition to developing Transum I am also the honorary Chair of a British Scouting Overseas District. In the end-of-year Bulletin I always contribute a puzzle and this year's is called Systematic Scouting. Can you solve all five levels?
There was a great deal written online last month about probably the best software program for impersonating humans ever released to the public. Such is its appeal that within days of its launch in November, ChatGPT attracted over a million people who logged in. I thought I would give it a try. I asked it to write a poem that I could include in this newsletter. Within the blink of an eye it came up with:
As the new year starts to dawn,
We look back on all that's gone,
But more importantly, we look ahead
To all the math problems we have yet to shed.
We'll tackle equations and solve equations,
We'll find the slope and the intersection,
We'll graph and we'll plot,
And we'll never give up or stop.
So let's make this new year our best yet,
With math skills that we won't forget,
We'll conquer every challenge with grace,
And leave behind any struggles or race.
So here's to a bright new year,
Filled with math and nothing to fear,
We'll work hard and we'll have fun,
And our math skills will be second to none.
I don't think that will win any prizes but it's fascinating nevertheless!
Finally the answer to last month's puzzle which was:
I am looking at the ornaments hanging on the Christmas tree (which are all one of three colours) and I notice that:
How many ornaments are on the tree and how many of each colour are there?
As I having been solving simultaneous equations for 80% of my life I cannot resist writing the given information as a set of three linear equations.
g + d = 8
r + d = 11
r + g = 13
Subtracting the first equation from the second reveals that r - g = 3
Adding this new equation to the third reveals that 2r = 16, so r = 8
Substituting this into the second equation gives 8 + d = 11, so d = 3
Substituting this into the first equation gives g + 3 = 8, so g = 5
Podcast listener Rick noticed that in the audio version of this Newsletter I failed to mention that the ornaments were all one of three colours. He goes on to say "let’s assume there are also some blue ornaments on the tree.
Let’s further assume there are 2 blue ornaments.
Solving now results in gold = 2, green = 4, blue = 2, and red = 7 for a total of 15 ornaments."
That's all for now,
John
P.S. You have to be odd if you want to be Number One.