Here are some exam-style questions on this statement:
- "The function \( f \) is defined by \( f(x) = \frac{5x + 5}{3x - 6} \) for \( x \in \mathbb{R}, x \neq 2 \)." ... more
- "The function \(f\) is defined by:" ... more
- "A function \(f\) is defined by \(f(x) = 2 + \dfrac{1}{3-x}, \text{ where } x \in \mathbb{R}, x \neq 3.\)" ... more
- "Let \(f(x) = \frac{9x-3}{bx+9}\) for \(x \neq -\frac9b, b \neq 0\)." ... more
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
A rational function is a function that can be represented as the quotient of two polynomials. The general forms of rational functions that are mentioned in the syllabus are:
$$ f(x) = \frac{ax + b}{cx^2 + dx + e} $$ and $$ f(x) = \frac{ax^2 + bx + c}{dx + e} $$
The first form represents a rational function where the numerator is a linear polynomial and the denominator is a quadratic polynomial. The second form represents a rational function where both the numerator and the denominator are polynomials of degree 2 and 1 respectively.
For the first form, an example could be:
$$ f(x) = \frac{2x + 3}{x^2 - 5x + 6} $$
For the second form, an example could be:
$$ f(x) = \frac{x^2 + 4x + 4}{3x + 6} $$
These functions can be graphed to visualize their behavior. It's important to note that the domain of a rational function is all real numbers except where the denominator equals zero, as this would make the function undefined.
If you use a TI-Nspire GDC there are instructions for finding asymptotes.
Quick Tips for Finding Asymptotes
Vertical Asymptotes: These occur where the function becomes undefined. To find vertical asymptotes, set the denominator of the rational function to zero and solve for \(x\). The values of \(x\) that make the denominator zero, without making the numerator zero at the same time, are the vertical asymptotes.
Horizontal Asymptotes: A horizontal asymptote describes the behavior of the graph as \(x\) approaches positive or negative infinity. To determine the horizontal asymptote, compare the degrees of the numerator and denominator:
- If the degree of the numerator is less than the degree of the denominator, the x-axis (y = 0) is the horizontal asymptote.
- If the degrees are the same, the horizontal asymptote is the ratio of the leading coefficients.
- If the degree of the numerator is greater than the degree of the denominator by one, there is no horizontal asymptote, and you should check for an oblique asymptote.
Oblique (or Slant) Asymptotes: An oblique asymptote occurs when the degree of the numerator is exactly one more than the degree of the denominator. To find the equation of the oblique asymptote, perform polynomial long division (see algebraic division below). The quotient (ignoring the remainder) gives the equation of the oblique asymptote.
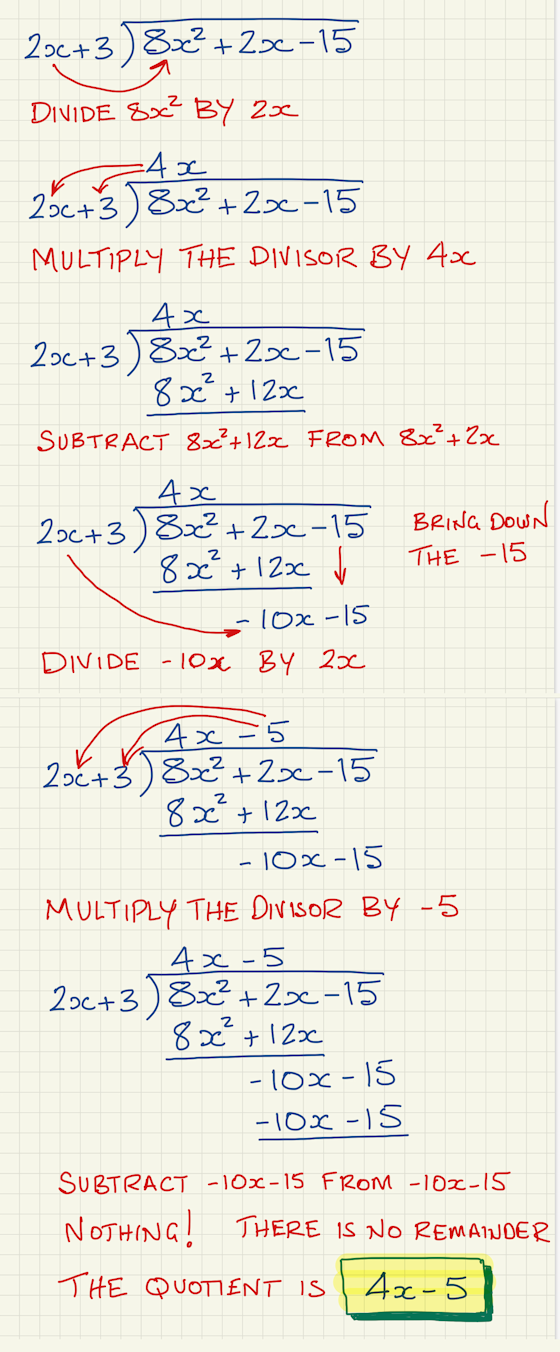
Algebraic division, often referred to as polynomial long division, is a method for dividing a polynomial by another polynomial of the same or lower degree. This technique is similar to long division used in arithmetic. The primary objective is to determine how many times the divisor fits into the dividend and to find the remainder if there is one. This method is particularly useful in simplifying rational expressions and solving polynomial equations.
The key formula for polynomial long division is as follows:
$$ \text{Dividend} = (\text{Divisor} \times \text{Quotient}) + \text{Remainder} $$Let's consider an example:
How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.

