Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.
 Differentiation Video A reminder of how to differentiate different types of functions and how to find the equations of tangents and normals.
Differentiation Video A reminder of how to differentiate different types of functions and how to find the equations of tangents and normals.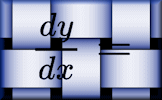 Differentiation Practise the technique of differentiating polynomials with this self marking exercise.
Differentiation Practise the technique of differentiating polynomials with this self marking exercise.
Here are some exam-style questions on this statement:
- "The table shows some values (rounded to one decimal place) for the function \(y=\frac{2}{x^2}-x, x\neq 0\).
\(x\) -3 -2 -1 -0.5 0.5 1 2 3 4 \(y\) 3.2 2.5 8.5 7.5 1.0 -2.8 (a) Complete the table of values.
" ... more - "Consider the graph of the function \(f(x)=7-3x^2-x^3\)" ... more
- "Consider the function \(f(x)=6 - ax+\frac 3{x^2},x\neq 0\)" ... more
- "Consider the function \(f\) defined by \(f(x)= \ln{(x^2 - 9)}\) for \(x > 3\)." ... more
- "The function \(f\) is defined for all \(x \in \mathbb{R}\). The line with equation \(y=5x+3\) is the tangent to the graph of \(f\) at \(x = 2\)" ... more
- "Consider the cubic function \(f(x)=\frac{1}{6}x^3-2x^2+6x-2\)" ... more
- "Consider the function \(f(x)=x^3-9x+2\)." ... more
- "Let \(f(x)=\frac{g(x)}{h(x)}\), where \(g(3)=36\), \(h(3)=12\), \(g'(3)=10\) and \(h'(3)=4\). Find the equation of the normal to the graph of \(f\) at \(x=3\)." ... more
- "Consider the functions" ... more
- "Consider the function \(f(x)=\frac{20}{x^2}+kx\) where \(k\) is a constant and \(x\neq0\)." ... more
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
In calculus, the tangent to a curve at a given point is the straight line that "just touches" the curve at that point. This means that the line goes through the point and its slope matches that of the curve at the given point. The slope of the tangent is equal to the derivative of the function at that point. The equation of the tangent line to the curve \( y = f(x) \) at the point \( (a, f(a)) \) is given by:
$$ y - f(a) = f'(a)(x - a) $$On the other hand, the normal to a curve at a given point is the straight line perpendicular to the tangent at that point. If the slope of the tangent is \( m \), then the slope of the normal is \( -\frac{1}{m} \). The equation of the normal line to the curve \( y = f(x) \) at the point \( (a, f(a)) \) is given by:
$$ y - f(a) = -\frac{1}{f'(a)}(x - a) $$Example 1 (Tangent): Find the equation of the tangent to the curve \( y = x^2 \) at the point \( (1,1) \).
First, we find the derivative of \( y = x^2 \), which is \( y' = 2x \). At \( x = 1 \), \( y' = 2 \). Using the point-slope form, the equation of the tangent is:
$$ y - 1 = 2(x - 1) \\ y = 2x - 1 $$Example 2 (Normal): Find the equation of the normal to the curve \( y = x^2 \) at the point \( (1,1) \).
Using the slope of the tangent which is 2, the slope of the normal is \( -\frac{1}{2} \). Using the point-slope form, the equation of the normal is:
$$ y - 1 = -\frac{1}{2}(x - 1) \\ y = -\frac{1}{2}x + \frac{3}{2} $$How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.

