Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.
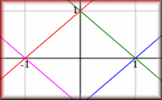 Graph Patterns Find the equations which will produce the given patterns of graphs.
Graph Patterns Find the equations which will produce the given patterns of graphs.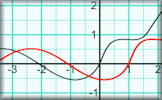 Transformations of Functions A visual aid showing how various transformations affect the graph of a function.
Transformations of Functions A visual aid showing how various transformations affect the graph of a function.
Here are some exam-style questions on this statement:
- "The graph of the curve A with equation \(y=f(x)\) is transformed to give the graph of the curve B with equation \(y=5-f(x)\)." ... more
- "(a) By completing the square, solve \(x^2+8x+13=0\) giving your answer to three significant figures." ... more
- "The graph of the following equation is drawn and then reflected in the x-axis" ... more
- "(a) Find the interval for which \(x^2 - 9x + 18 \le 0\)" ... more
- "(a) Write \(2x^2+8x+27\) in the form \(a(x+b)^2+c\) where \(a\), \(b\), and \(c\) are integers, by 'completing the square'" ... more
- "Let \(f (x)=a(x-b)^2+c\). The vertex of the graph of \(f\) is at (4, -3) and the graph passes through (3, 2)." ... more
- "A function is defined as \(f(x) = 2{(x - 3)^2} - 5\) ." ... more
- "\(f\) and \(g\) are two functions such that \(g(x)=3f(x+2)+7\)." ... more
- "Let \(f(x)=5x^2-20x+k\). The equation \(f(x)=0\) has two equal roots." ... more
- "Let \(f(x) = {x^2}\) and \(g(x) = 3{(x+2)^2}\) ." ... more
- "Part of the graph of a function, \(f\) , is shown in the following diagram. The graph of \(y = f(x)\) has a y-intercept at \((0, 1.5)\) , an x-intercept at \((a , 0)\) and horizontal asymptotes \(y = 5\) and \(y = -2\)." ... more
- "Let \(f\) and \(g\) be functions such that \(g(x) = 3f(x - 2) + 7\) ." ... more
- "The graph of \( y = f(|x|) \) for \( -2 \leq x \leq 2 \) is shown in the following diagram." ... more
- "Two functions are defined as follows: \(f(x) = 2\ln x\) and \(g(x) = \ln \frac{x^2}{3}\)." ... more
Click on a topic below for suggested lesson Starters, resources and activities from Transum.
Furthermore
In the study of functions, understanding graph transformations is crucial for interpreting and predicting the behaviour of different equations. A transformation modifies the appearance of the original graph, which can be translated, reflected, stretched, or compressed.
Translations involve shifting the graph without changing its shape. The function \( y = f(x) + b \) results in a vertical translation by \( b \) units. If \( b \) is positive, the shift is upwards; if negative, the shift is downwards. Conversely, \( y = f(x + a) \) translates the graph horizontally by \( a \) units to the left for positive \( a \), and to the right for negative \( a \).
Reflections flip the graph over a specific axis. Reflecting over the x-axis, \( y = -f(x) \), inverts the graph upside-down. Reflecting over the y-axis, \( y = f(-x) \), produces a mirror image of the graph.
Vertical stretches are achieved by multiplying the function by a scale factor \( p \), denoted as \( y = pf(x) \). The graph stretches away from the x-axis if \( p > 1 \) or compresses towards it if \( 0 < p < 1 \).
Horizontal stretches are expressed by \( y = f(qx) \), where \( 1/q \) is the scale factor. The graph stretches horizontally away from the y-axis for \( q < 1 \) and compresses towards it for \( q > 1 \).
Composite transformations involve performing more than one transformation on a graph. It's essential to note the order in which transformations are applied, as it sometimes affects the outcome.
Let's consider an example. To apply a vertical stretch by a factor of 3 combined with a horizontal shift 2 units to the right, the transformation would be represented as:
$$ y = 3f(x - 2) $$
This indicates that every point on the graph of \( y = f(x) \) is first moved 2 units to the right, and then stretched vertically away from the x-axis by a factor of 3.
This video on Transformation of Functions is from Revision Village and is aimed at students taking the IB Maths AA Standard level course
How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.

