International Baccalaureate Mathematics

Syllabus Content

Concept of a function, domain, range and graph. Function notation, for example f(x), v(t), C(n). The concept of a function as a mathematical model. Informal concept that an inverse function reverses or undoes the effect of a function. Inverse function as a reflection in the line y=x, and the notation f^-1(x)

Here are some specific activities, investigations or visual aids we have picked out. Click anywhere in the grey area to access the resource.

Functions An online exercise on function notation, inverse functions and composite functions.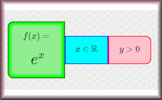

Domain, Range and Asymptotes Match the functions with their features. A drag and drop activity.

Here are some exam-style questions on this statement:

"(a) A function is represented by the following function machine." ... more

"Here is a function machine that produces two outputs, A and B." ... more

"The circumference of a given circle $C$ can be represented by the function $C(A) =2\sqrt{A \pi}, A \ge 0 $ where $A$ is the area of the circle. The graph of the function $C$ is shown for $0 \le A \le 16$." ... more

"The diagram shows the graph of $y=f(x)$, for $-3\le x \le 4$." ... more

"The circumference of a given circle $C$ can be represented by the function $C(A) = 2 \sqrt{A \pi}$ , $A \ge 0 $ , where $A$ is the area of the circle. The graph of the function $C$ is shown for $0 \le A \le 10$." ... more

"Consider the function $f(x) = k^x $ where $x, k \in \mathbb{R}$ and $ x \gt 0, k > 1$." ... more

"A function $ g $ is defined by $ g(x) = \frac{3x - 10}{x^2 - 9} $, where $ x \in \mathbb{R} $, $ x \neq \pm3 $." ... more

See all these questions

Here is an Advanced Starter on this statement:

Catering for a Function
Find f(x) given f(x-1). more

Click on a topic below for suggested lesson Starters, resources and activities from Transum.

Functions A relationship between two sets can be called a mapping. Elements of the first set (domain) are mapped to elements of the second set (range). A function is a special type of mapping for which one value in the domain maps to one, and only one value in the range.Pupils in Primary school will use the concept of function machines to perform calculations. They will then learn to ‘work backwards’ to find the inverse function. The study of functions becomes more formal as pupils become more proficient and able to cope with more complex mathematical ideas.

Furthermore

Official Guidance, clarification and syllabus links:

Example: $f(x)=\sqrt{2-x}$, the domain is $x\le 2$, range is $f(x)\ge 0$.

A graph is helpful in visualising the range.

Example: Solving $f(x)=10$ is equivalent to finding $f^{-1}(10)$.

Students should be aware that inverse functions exist for one to one functions; the domain of $f^{-1}(x)$ is equal to the range of $f(x)$.

In mathematics, a function is a relationship between a set of inputs and a set of permissible outputs. The domain of a function is the set of all possible inputs, while the range is the set of all possible outputs. Function notation, such as $ f(x) $, is used to denote a function $ f $ applied to an input $ x $. The graph of a function is a visual representation of this relationship, plotting inputs versus outputs.

An inverse function reverses the effect of a given function. If a function $ f $ takes an input $ x $ and produces an output $ y $, then its inverse $ f^{-1} $ takes $ y $ as input and returns $ x $ as output. Graphically, the inverse function is a reflection in the line $ y = x $.

Key Formulae:

$$ \begin{align*} \text{Function: } & f(x) \\ \text{Domain: } & \{x \,|\, \text{conditions on } x\} \\ \text{Range: } & \{f(x) \,|\, x \in \text{Domain of } f\} \\ \text{Inverse Function: } & f^{-1}(y) \text{ such that } f(f^{-1}(y)) = y \end{align*} $$

Example:

$$ \begin{align*} \text{Let } f(x) &= x^2, \text{ for } x \geq 0 \\ \text{Domain: } & [0, \infty) \\ \text{Range: } & [0, \infty) \\ f^{-1}(y) &= \sqrt{y}, \text{ for } y \geq 0 \\ \text{Thus, } f^{-1}(f(x)) &= f^{-1}(x^2) = \sqrt{x^2} = x \end{align*} $$

If you use the TI-Nspire calculator you can find instructions for defining a function on the GDC Essentials page. Ver useful if you need to evaluate the function for different x-values and plot a graph of the function.

This video on Functions: Overview and Types is from Revision Village and is aimed at students taking the IB Maths AI Standard level course.

This video on Domain, Range, Composite, Inverse is from Revision Village and is aimed at students taking the IB AA Maths Standard level course

How do you teach this topic? Do you have any tips or suggestions for other teachers? It is always useful to receive feedback and helps make these free resources even more useful for Maths teachers anywhere in the world. Click here to enter your comments.