
 |
Exam-Style Questions.Problems adapted from questions set for previous Mathematics exams. |
1. | GCSE Higher |
Find the exact value of tan45° × sin30° × cos30°
Give your answer in its simplest form.
2. | GCSE Higher |
(a) Write down the exact value of \(\tan 60^o\).
(b) Find the exact area of this triangle.

3. | GCSE Higher |

The area of triangle ABC (not drawn to scale) is
$$ \frac{35 \sqrt{3}}{4} m^2$$If AB = \(x+2\) metres and AC = \(2x+1 \) metres, find the value of \(x\).
4. | IB Analysis and Approaches |
The function \(f\) is defined by \(f(x) = \cos{ax} \) , where \(a > 0\). The following diagram shows part of the graph of \(f\), where x is in radians. There are local maxima at \(x = b , 2b \text{ and } 3b\).
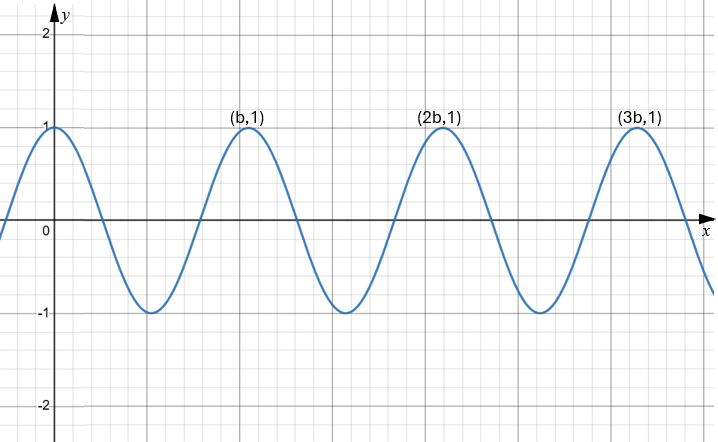
(a) Find an expression for \(b\) in terms of \(a\).
The function \(g\) is defined by \(g(x) = 2\cos{ \dfrac{bx}{3}} \)
(b) On the axes above, sketch the graph of \(g\).
5. | IB Standard |
The diagram shows part of the graph of \(y=a\sin{(bx)}+c\) with a minimum at \((-2.5,-2)\) and a maximum at \((2.5,4)\).
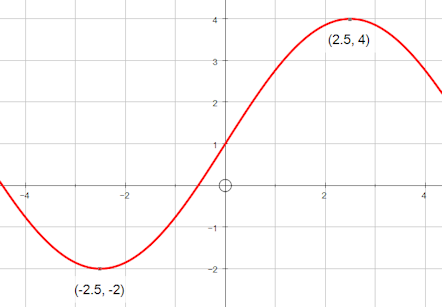
(a) Find \(a\).
(b) Find \(b\).
(c) Find \(c\).
6. | IB Standard |
A Big Wheel at an amusement park has a diameter of length 70 metres which rotates at a constant speed. The bottom of the wheel is h metres above the ground. A seat starts at the bottom of the wheel.The wheel completes one revolution in 4 minutes. [The diagram is not to scale]

(a) After 2 minutes, the seat is 76m above the ground. Find h.
(b) After t minutes, the height of the seat above ground is given by \(f(t) =41+ k \cos{ \frac{\pi t}{2}} \) for \(0 \le t \le 16 \).
Find the value of k.
(c) Find when the seat is 40 m above the ground for the third time.
7. | IB Standard |
(a) Sketch the graph of \(f(x) = 4\sin x - 5\cos x \), for \(–2\pi \le x \le 2\pi \).
(b) Find the amplitude of \(f\).
(c) Find the the period of \(f\).
(d) Find the \(x\)-intercept that lies between 0 and 3.
(e) Hence write \(f(x)\) in the form \(a \sin (bx + c) \).
(f) Write down one value of \(x\) such that \(f'(x) = 0 \).
(g) Write down the two values of \(p\) for which the equation \(f(x) = p\) has exactly two solutions.
8. | IB Analysis and Approaches |
Consider a function \(f\), such that \(f(x)=7.2\sin(\frac{\pi}{6}x + 2) + b\) where \( 0\le x \le 12\)
(a) Find the period of \(f\).
The function \(f\) has a local maximum at the point (11.18,10.3) , and a local minimum at (5.18.-4.1).
(b) Find the value of b.
(c) Hence, find the value of \(f(7)\).
A second function \(g\) is given by \(g(x)=a\sin(\frac{2\pi}{7}(x -4)) + c\) where \(0 \le x \le 10\)
The function \(g\) passes through the points (2.25,-3) and (5.75,7).
(d) Find the value of \(a\) and the value of \(c\).
(e) Find the value of x for which the functions have the greatest difference.
9. | IB Analysis and Approaches |
The height of water in metres above Chart Datum* in the Port of Bristol on a day in January is modelled by the function:
$$H(x) = a \sin(b(x-c)) +d$$where \(x\) is the number of hours after midnight GMT, and \(a,b,c, \text{ and } d \) are constants greater than zero.
The following graph shows the height of the water for 19 hours starting at midnight.

The first low tide occurs at 02:23 and next low tide occurs at 14:28.
Throughout the day the height of the water fluctuates between 1m and 13.8m.
(a) Show that \(a = 6.4\).
(b) Find the value of \(b\) to two decimal places.
(c) Find the value of \(d\).
(d) Find the smallest possible value of \(c\) to one decimal place.
(e) Using the values of \(a,b,c, \text{ and } d \) found above, find the height of the water at 10:00.
(f) Determine the number of hours, during this January day, for which the tide is higher than 10 metres above Chart Datum.
* Chart Datum is a reference datum or level from which heights and depths on a nautical chart are measured. Chart Datum is determined by the lowest astronomical tide, which is the level of the sea at its lowest possible level. It is also the reference level used to measure the depth of water in a harbour or other body of water.
If you would like space on the right of the question to write out the solution try this Thinning Feature. It will collapse the text into the left half of your screen but large diagrams will remain unchanged.
The exam-style questions appearing on this site are based on those set in previous examinations (or sample assessment papers for future examinations) by the major examination boards. The wording, diagrams and figures used in these questions have been changed from the originals so that students can have fresh, relevant problem solving practice even if they have previously worked through the related exam paper.
The solutions to the questions on this website are only available to those who have a Transum Subscription.
Exam-Style Questions Main Page
To search the entire Transum website use the search box in the grey area below.