Graph Patterns :: A Coordinated Challenge

The equation of any straight line can be written in the form:

y = mx + c

where m is the gradient of the line and c is the y-coordinate of the point where the line crosses the y-axis (y-intercept).

The gradient of the line can be found by considering to points on the line where the exact coordinates can be determined. The rise is the vertical distance between these points and the run is the hirizontal distance. The gradient is the rise divided by the run (rise over run).

If the line slopes from bottom left to upper right the gradient is positive. If the line slopes from top left to bottom right the gradient is negative.

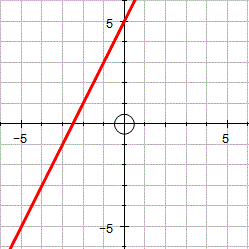For example the graph shown to the left has a gradient of 2 (for every one square across the line goes up two squares. Rise over run is 2 over 1 which is 2.) The y-intercept is 5 so the equation is:

y = 2x + 5

A horizontal line with a y-intercept of three has equation:

y = 3

A vertical line with an x-intercept of three has equation:

x = 3